Spontaneous oscillations in non-linear networks
 We study a model for flow networks with edges that display non-linear conductance and with nodes that allow for internal accumulation/depletion of volume. We observe emerging dynamics in the form of self-sustained waves which travel through the system under constant boundary conditions. These spontaneous fluctuations persist after drastically changing the topology of the network and the boundary conditions. We show that the dependence of the frequency of the spontaneous fluctuations on different changes of the structure of the network can be explained by a unique topological measure.
We study a model for flow networks with edges that display non-linear conductance and with nodes that allow for internal accumulation/depletion of volume. We observe emerging dynamics in the form of self-sustained waves which travel through the system under constant boundary conditions. These spontaneous fluctuations persist after drastically changing the topology of the network and the boundary conditions. We show that the dependence of the frequency of the spontaneous fluctuations on different changes of the structure of the network can be explained by a unique topological measure.
(in preparation)
Phenotypes of Fluctuating Flow
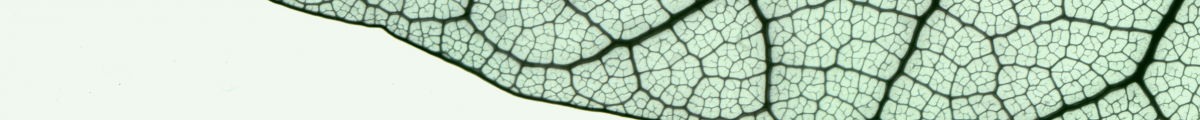
Complex distribution networks are pervasive in biology. Examples include nutrient transport in the slime mold Physarum polycephalum as well as mammalian and plant venation. Adaptive rules are believed to guide development of these networks and lead to a reticulate, hierarchically nested topology that is both efficient and resilient against perturbations.
 However, as of yet no mechanism is known that can generate such networks on all scales. We show how hierarchically organized reticulation can be generated and maintained through spatially collective load fluctuations on a particular length scale. We demonstrate that the resulting network topologies represent a trade-off between optimizing power dissipation, construction cost, and damage robustness and identify the Pareto-efficient front that evolution is expected to favor and select for. We show that the typical fluctuation length scale controls the position of the networks on the Pareto front and thus on the spectrum of venation phenotypes. We compare the Pareto archetypes predicted by our model with examples of real leaf networks.
However, as of yet no mechanism is known that can generate such networks on all scales. We show how hierarchically organized reticulation can be generated and maintained through spatially collective load fluctuations on a particular length scale. We demonstrate that the resulting network topologies represent a trade-off between optimizing power dissipation, construction cost, and damage robustness and identify the Pareto-efficient front that evolution is expected to favor and select for. We show that the typical fluctuation length scale controls the position of the networks on the Pareto front and thus on the spectrum of venation phenotypes. We compare the Pareto archetypes predicted by our model with examples of real leaf networks.
https://arxiv.org/abs/1707.03074
Global Optimization, Local Adaptation, and the Role of Growth in Distribution Networks
Highly optimized complex transport networks serve crucial functions in many man-made and natural systems such as power grids and plant or animal vasculature. Often, the relevant optimization functional is nonconvex and characterized by many local extrema. In general, finding the global, or nearly global optimum is difficult. In biological systems, it is believed that such an optimal state is slowly achieved through natural selection. However, general coarse grained models for flow networks with local positive feedback rules for the vessel conductivity typically get trapped in low efficiency, local minima. We show how the growth of the underlying tissue, coupled to the dynamical equations for network development, can drive the system to a dramatically improved optimal state. This general model provides a surprisingly simple explanation for the appearance of highly optimized transport networks in biology.
models for flow networks with local positive feedback rules for the vessel conductivity typically get trapped in low efficiency, local minima. We show how the growth of the underlying tissue, coupled to the dynamical equations for network development, can drive the system to a dramatically improved optimal state. This general model provides a surprisingly simple explanation for the appearance of highly optimized transport networks in biology.
https://journals.aps.org/prl/pdf/10.1103/PhysRevLett.117.138301
Structural Self-Assembly and Avalanchelike Dynamics in Locally Adaptive Networks
Many biological transport networks employ adaptive strategies to respond to stimuli, damage, and other environmental changes. We model these adapting network architectures using a generic dynamical system on weighted graphs and find in simulation that these networks ultimately develop a hierarchical organization of the final weighted architecture accompanied by the formation of a system-spanning backbone. In addition, we find that the long term equilibration dynamics exhibit behavior reminiscent of glassy systems characterized by long periods of slow changes punctuated by bursts of reorganization events.
https://journals.aps.org/pre/pdf/10.1103/PhysRevE.92.012801